THE OFFICE OF THE PRESIDENT, REGIONAL ADMINISTRATION AND LOCAL GOVERNMENT.
SECONDARY EXAMINATION SERIES
MARCH 2025
MATHEMATICS FORM FOUR
TIME: 2:30HRS
Instructions
- This paper will consist of sections A and B with a total of 14 questions
- Answer all questions in both sections A and B
- Section A will carry 60 marks and section B will carry 40 marks
- Mathematical tables/ Calculator may be used
- Write your Examination number on the top right corner of your answer booklet provided. Use R = 6400 and π= 3.14
SECTION A (60 MARKS)
1. (a) Write a number in which O represents hundreds, 7 represents ten thousands, 3 represents ones, 9 represents thousands, 6 represents hundred thousand and 8 represents tens.
(b) Convert and 360% to decimal, then approximate their products to one significant figure.
(c) 14 - [−2 − (8 ÷ 2) + 5]
2. (a) Express (7 + √2)2 in the form of a + b√c
(b) Find the value of x given that 2logx = log4 + log (2x − 3)
(c) Solve for y given that 125y+1 + 53y = 630
3. (a) In a class of 60 students, 40 students like History, 36 students like Geography, 24 students like both subjects. Find the number of students who like;
(i) history only
(ii) geography only
(iii) either history or geography
(iv) neither history nor geography
(b) When a fair die is tossed, find the probability that the number obtained is,
(i) more than five
(ii) at least one
(iii) at most six.
4. (a) In the figure below AB= 3cm, < BCA = 300 and <BDC = 90. Given that <ABC =<BDC
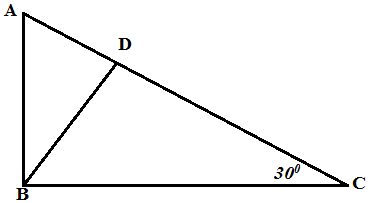
(i) Prove that ∆BDC ~ ∆ ABC
(ii) Calculate length BD
(b) Two concentric regular Hexagons are such that the radius of circle inscribing the larger on is twice that inscribing the smaller hexagon. If the radius of smaller is 5cm. find area between the two Hexagons.
5. (a) The value of “V” of a diamond is proportional to the square of its weight “W”. It is known that a Diamond weighing 10grams is worth Tsh 200,000/=
(i) Write down expression that relates V and W
(ii) Find the weight of a diamond that worth Tsh 5,000,000/=
(b) A day worker is paid Tsh 48,000/= for 16 hours work.
(i) What is the rate of payment?
(ii) At this rate, how much would he receive for 30 hours of work?
(iii) At this rate, for how long must he work in order to receive Tsh 120,000/=
6. (a) A students had two plant seedlings. She measured the rate at which the seedlings were growing. Seedling A grew 5 cm in 10 days and seedling B grew 8 cm in 12 days. Which seedling was growing more quickly?
(b)Suppose x varies directly as y square and inversely as p. if x=2, when y=3 and p=1, Find the value of y when x=4 and p=5.
7. (a) Kabujage Saved Tshs 6 million in a savings Bank whose interest rate was 100% compounded annually. Find the amount in Kabujages’s savings accounts after 5 years
(b) A small business sells products with 1,000,000tshs during its first year. The owner of the business has set a goal of increasing annual sales by Tshs 750,000 each year. Assuming this goal is met. Find the total sales during the first 10 years of the business in operation
8. a) Given that (Z + 1) is directly proportional to x and inversely proportional to the square root of y. If x =2 when y=4 and Z =4 find Z when X = 3 and y =9
(b) A bus travels 240 km using 16 litres of diesel. How many liters of diesel are needed to drive 90km?
9. (a) Solve the quadratic equation x2 – 8x +7 = 0
(b) A field is 10m longer than its wide. The area is 7,200m2. What is the width?
10. (a)In a class of 20 pupils, 12 pupils study English but not History, 4 pupils study History but not English and one pupils study neither English nor History. How many pupils in class study History? (Use Venn diagram)?
(b)Inside the class, there are 6 wooden chairs and 8 plastic, two chairs are taken at random from the class without replacement. By using tree diagram, find the probability that the first chair taken is wooden and the second chair is plastic.
FORM FOUR MATHEMATICS EXAM SERIES 207
FORM FOUR MATHEMATICS EXAM SERIES 207
PRESIDENT’S OFFICE, REGIONAL ADMINISTRATION AND LOCAL GOVERNMENT
SECONDARY EXAMINATION SERIES,
MID TERM ONE – MARCH-2024
MATHEMATICS FORM FOUR
Time: 3Hours
Instructions
- This paper consists of sections A and B with a total of fourteen (14) questions.
2. Answer all questions in sections A and B
3. Each question in section A carries six (6) marks while each question in section B carries ten (10) marks.
4. All necessary working and answers for each question must be shown clearly.
5. NECTA Mathematical tables and non-programmable calculator may be used.
6. All communication devices and any unauthorized materials are not allowed in the examination room.
7. Write your examination number on every page of your answer sheet(s).
SECTION A. (60 MARKS)
Answer all questions in this section.
- (a) Given the number 857636721. Write this number
- To the nearest thousands
- To hundreds
- In expanded form
(b) Three brothers visited their grandfather at interval of 5 days, 7 days and 12 days. If they started together on 15th July. Find the date they will visit the grandfather together next time. (Each month has 30days)
- (a) (i) Solve for X if
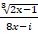 =16
=16
(ii) Simplify ![]()
(b) given that log 34 =1.262 and log 35=1.1465. Find log 3 0.8
- (a)In a class of 60 students, 40 students like history, 36 like geography, 24 students like both subjects. Find the number of students who like
- History only
- Either history or geography
- Geography only
- Neither history nor geography
(b) When a fair die is tossed, find probability that the number obtained is
- More than five
- At least one
- At most six
- If a=5i+4j, b= -3i+3j, and c = -2i+5j. find
- V= 3a+b-3c
- The magnitude of V
(b) given the point A (3,3) B (-3,1) C (-1,-1) and D (1,-7)
- Show the line through AB and CD are perpendicular to each other.
- Find the equation of the line through point (-4,5) which is parallel to BC
- (a) A school cow produces18 litres of milk every day. How many cows of same type should the school keep to get 126 litres of milk every day.
(b) One family from England traveled for holiday to France and exchanged 450 pounds for Euros when exchange rate was 1.41 Euros to Pound. They spent 500 Euros and then exchanged the remaining amount into pounds by that time the exchange rate had become 1.46 Euros to Pound. How much money remained in terms of pounds?
- (a) A school hall has 32 rows of seats. If there are 26 seats in the first row, 30 seats in 2nd row, 34 seats in 3rd row and so on. How many seats are there in a theatre?
(b) Mr Cuthbert starts an employment with a monthly salary of 340,000 and receives an increment of Tsh 12,000/= per year.
- What will be his salary in fourteen year of employment
- After how long will he earning Tsh 592,000 per month.
- (a) The fifth and eleventh terms of AP are 8 and -34 respectively. Find the sum of first ten terms.
(b) A school wishes to invest Tsh 100,000,000 in a bank which pays an interest rate of 2% compounded annually.
- Find total amount of money that will accumulate after 2years
- Calculate the interest after 2 years
- (a) Asha is three years older than her brother Juma. Three years to come the product of their age will be 130 years.
- Formulate the quadratic equation representing information above
- Use the quadratic equation formula to find present age of Asha and Juma
- Solve the equation by completing the square
2x2-3x-5=0
- What do the following terms mean as used in Accounts
- Cash book
- Asset
- Credit transactions
(b) A company bought two cars for Tsh 25,000,000/= each. If one car was sold at a profit of 18% and another was sold at a loss of 6%. In the whole transaction there was no loss. What was the profit made by the company?
- If the square of the hypotennce of an isosceles right angled Triangle is 128cm2. Find the length of each other sides.
(b) From the top of the tower, of height 60m the angle of depression of the top and the bottom of a building are observed to be 300 and 600 respectively. Find height of the building.
SECTION B. 40 MARKS
- (a) If matrix A is singular, what will be value of Y given that
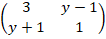
(b) Solve the following simultaneous equation by matrix method
2x+y=7
4x+3y=17
(c) Find the image of (3,5) after rotation of 2700 about the origin in the ant-clockwise direction.
- (a) A rectangular box with top WXYZ and base ABCD has AB= 9 cm, BC =12 cm, WA=3cm. calculate
- The length of AC
- The angle between WC and AC
- Consider the following frequency distribution table below.
| Marks | 0-9 | 10-19 | 20-29 | 30-39 | 40-49 | 50-59 | 60-69 | 70-79 | 80-89 | 90-99 | 100-109 | 110-119 |
| frequency | 1 | 2 | 5 | 11 | 21 | 20 | 17 | 10 | 6 | 4 | 2 | 1 |
(a)Draw the histogram and use it to estimate the mode in one decimal place
(b) Find the value of angle x in the figure below
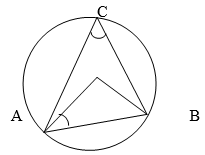
- (a) A function F is defined by the formula f(x) =
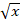 where x is a whole number.
where x is a whole number.
- If f(x) =25. Find the value of x
- Fund the value of

(b)A craftsman wishes to decide how many of each type A and B charcoal stove has to fabricate in order to maximize profit for the month. Unit profit for type A stove is 1000/= and 1500/= for type B. type A stove requires 1m2 of mild steel sheet per unit and type B 2m2 . He has only 12m2 of mild steel available. He can fabricate a total of 8 stoves of either type per month. How many stove of each type should be fabricated.
FORM FOUR MATHEMATICS EXAM SERIES 183
FORM FOUR MATHEMATICS EXAM SERIES 183
PRESIDENT�S OFFICE
REGIONAL ADMINISTRATION AND LOCAL GOVERNMENT
SECONDARY EXAM SERIES
FORM 4 BASIC MATHEMATICS
SECTION A (60 MARKS)
Answer ALL questions in this section
1(a)Express the number 0.000038583
- In standard form
- Correct to 4 significant figures
(b)Change ![]() into fraction
into fraction
2(a)Solve the following equation simultaneously:
![]()
(b)Rationalize the denominator of the expression ![]()
3(a)Solve the following equations simultaneously
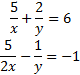
(b)Given ![]() . Find:
. Find: ![]()
4(a)Find the equation of the perpendicular bisector of the points A(4,8) and B (-4,-6)
giving your answer in the form ![]()
(b)Given that ![]() .
.
Find the relation between the three vectors a, b and c
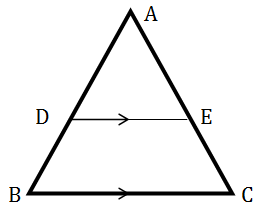
5. In the figure below ![]() //
//![]() and
and ![]()
If the area of DECB is 21cm2; find the area of ![]()
6(a) Given that w is directly proportional to x2 and inversely proportional to t and that
w=12 when x=2 and t=2. Find the value of w when x=3 and t=3
(b)Sophia and Alex had each Tsh.10,000. If Sophia wanted to buy the South African Rand and Alex wanted to buy the Malawian Kwacha, how much would each one receive?
(1 Rand =210 Tanzania shillings; and 1 Malawian Kwacha=10.80 Tanzanian shillings)
7(a)Given that A:C=10:7 and B:C=5:14; Find A:B
(b)Anna paid Tsh. 20,000 for 10 books. She sold ![]() of them at Tsh. 3,000 each and the remaining at Tsh. 3,500each. What was her percentage Loss or percentage profit?
of them at Tsh. 3,000 each and the remaining at Tsh. 3,500each. What was her percentage Loss or percentage profit?
8.(a)The sum of three terms which are in G.P is 28 while the product of these terms is 512.
Find the largest term.
(b)The fourth and sixth terms of an arithmetic progression are 45 and 55 respectively. Find;
- The first term
- The tenth term
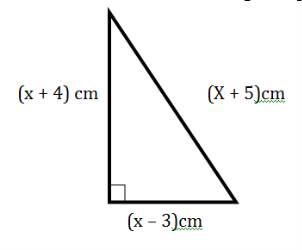
9.(a)Find value of x in the following triangle and hence find the area of the triangle.
(b)It is known that ![]() , find the relationship between
, find the relationship between ![]()
10(a)Factorize![]()
(b)Find the only solution of the equation ![]()
SECTION B (40 MARKS)
Answer any four (4) questions from this section
11(a)The following graph shows the feasible region of a linear programming problem where the shaded region is the feasible region. Study the graph and answer the questions that follow.

- Write the coordinates of Corner points A, B, C, D, E, and F
- Write the equations represented by the letters and hence the corresponding inequalities of
- Find the minimum value given that
12. The following frequency distribution table shows scores of marks of 50 students in a Mathematics Test:
| CLASS INTERVAL | 1.0 � 2.0 | 2.0 � 3.0 | 3.0 � 4.0 | 4.0 � 5.0 | 5.0 � 6.0 | 6.0 � 7.0 |
| FREQUENCY |
Calculate the measures of central Tendency
13(a) Town X and Y are located at (60�N, 30�E) and (60�N, 45�W) respectively on the earth�s surface. Calculate the distance between the two towns in Kilometers.
(b)Find the value of the angles marked X and Y in the figure below, given that O is the center of the circle.
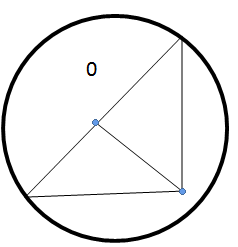
(c)Find the area of a prism (rectangular) with l=8cm, w=6cm and h=4cm
14.from the balances given below, prepare a balance sheet at 31st December 2010. Capital shs 205,000; Furniture shs.54,000; cash in hand shs 16,000; Net profit sh.74,000; Motor van sh 30,000; stock sh. 110,000; Drawings shs. 24,000; shop fittings shs 20,000; loan from Bank sh. 80,000; Debtors shs. 180,000; Creditors shs.45,000 and Bank Overdraft shs. 30,000
15.(a)Use the inverse of matrix B to find matrix A given that;
![]()
(b)Write two conditions fr a transformation to be a linear.
(c)By using a sketch and not otherwise, find the image of P(3, 4) when rotated about 90� anticlockwise followed by another rotation of 180� clockwise.
16.(a)The ordered pairs of a Quadratic function f are ![]() Find the function f(x)
Find the function f(x)
(b)A fair die is tossed once. Find the probability that an even number or a prime number occurs
(c) Given that ![]()
1
FORM FOUR MATHEMATICS EXAM SERIES 82
FORM FOUR MATHEMATICS EXAM SERIES 82
Student’s Examination No.....................................
THE PRESIDENT’S OFFICE
MINISTRY OF EDUCATION, REGIONAL ADMINISTRATION AND LOCAL GOVERNMENT
SECONDARY EXAMINATION SERIES
MATHEMATICS MID TERM EXAMINATION-MARCH
FORM FOUR-2021
Time: 3Hours
Instructions.
- This paper consists of section A, and B with a total of 14 questions
- Answer all questions in section A and B.
- Each question in Section A carries 06 marks, while each question in section B carry 10 marks
- All necessary working and answers for each question must be shown clearly.
- NECTA mathematical tables and non programmable calculators may be used.
- Cellular phones and any unauthorized materials are not allowed in the examination room.
- Write your number on every page of your answer booklet.
SECTION A (20 Marks)
Answer All questions in this section.
- (a) Write;
- 4.20098 into two decimal places
- 0.002758 into two significant figures
- 0.0497 rounding off to hundredth
(b) Use mathematical tables to evaluate ![]()
- (a) Solve for

(b) Evaluate ![]() without using mathematical tables
without using mathematical tables
- (a) Two sets A and B are subsets of a given universal set µ =
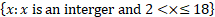 Find
Find
(b) A mother’s age is four times the age of her daughter. If the sum of their ages is 50 years, find the age of the mother.
- (a) Given that
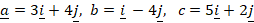
Find the magnitude of ![]()
Leaving your answer in the form of ![]()
(b) Find the equation of the line passing at the point (6,-2) and it is
perpendicular to the line crosses the ![]() – axis at 3 and the
– axis at 3 and the ![]() – axis at -4
– axis at -4
- (a) The ratio of the areas of two similar polygons is 144:225. If the length of a side of the small polygon is 60cm, find the length of the corresponding side of the other polygon.
(b) Find the length of a side and the perimeter of a regular nonagon inscribed in a circle of radius 6cm
- (a) The variable
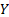 is directly proportional to
is directly proportional to 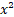 and inversely proportional to
and inversely proportional to 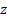 . If
. If 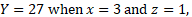 find
find 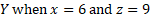
(b) A car is travelling steadily covers a distance of 480km in 25 minutes. What is its rate in ![]()
- (a) a car was bought for 4,000,000/= and sold for 4,500,000. Calculate
- The profit made
- The percentage profit
(b) A factory employs skilled, semi-skilled and office workers in the ration 6:5:4 respectively. If there are 120 semi-skilled workers, how many skilled workers are there?
- (a) The sum of the first six terms of an A.P is 72 and the second term is seven times the fifth term. Find the sum of the first ten terms of this A.P
(b) Find the amount accumulated at the end of 2 years after investing 500,000/= at a compound interest rate of 10% annually.
- (a) Without using tables, evaluate
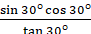
(b) a ladder reaches the top of a vertical wall 18m high when the other end on the ground is 8m from the wall. Find the length of the ladder correct to one decimal place
- (a) Solve the equation
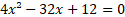 by using quadratic formula.
by using quadratic formula.
(b) Pulukuchu is 6 years younger than her brother Mpoki. If the product of their age is 135, find how old is Pulukuchu and Mpoki
SECTION B (40 MARKS)
- A small industry makes two types of clothes namely type A and type B. Each type A take 3 hours to produce and uses 6 meters of material and each type B take 6 hours to produce and uses 7 meters of material. The workers can work for a total of 60 hours and there is a 90 meters of materials available. If the profit on a type a cloth is 4,000 shillings and on type b Is 6,000 shillings, find how many each.
- The following distribution table shows the scores of 64 students in a chemistry weekly test;
| Scores | 30-39 | 40-49 | 50-59 | 60-69 | 70-79 | 80-89 | 90-99 |
| Frequency | 5 | 10 | 15 | 17 | 4 | 6 | 7 |
- Calculate the mean and mode (do not us assumed mean)
- Draw the give and use it to estimate the median
- (a) Calculate the distance from Chagwe (5?S, 39?E) to Minga (12?S,39?E) in kilometres. Use π = 3.14, and th radius of the earth R = 6370 km and write the answer correct to 1 decimal place.
(b) If a bus leaves Chagwe at 8.00 am on Monday and travels at 40km/hour, at what time will it reach Minga?
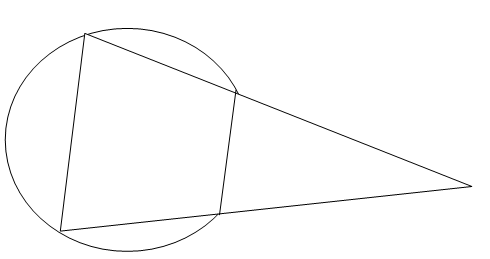
(c) Find the values of ![]() in the figure below;
in the figure below;
- Study the following trial balance and then answer the questions that follow:

NB: Closing stock was Tshs 7,400;
Prepare:
- Trading profit and loss account
- Balance sheet
- (a) Find the inverse of matrix
A![]()
(b) Use the result of part (a) to solve the simultaneous equation;
![]()
(c) Find the value of ![]() which the matrix
which the matrix ![]() has no inverse
has no inverse
- (a) The function
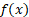 is defined by
is defined by
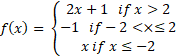
- Sketch the graph of
- State the domain and range of
(b) The probability that Anna and John will be selected for advanced level is 0.5 and 0.3 respectively. Determine the probability that;
- Both of them will not be selected
- Anna will be selected and John will not be selected
- One of them will be selected
1 | Page
FORM FOUR MATHEMATICS EXAM SERIES 44
FORM FOUR MATHEMATICS EXAM SERIES 44
THE PRESIDENT’S OFFICE MINISTRY OF EDUCATION AND VOCATIONAL TRAINING
MID TERM EXAMIATIONS
024 MATHS- FOUR
Duration: 3 Hours
INSTRUCTIONS.
- This paper consists of two sections A and B.
- Answer all questions in Section A and only four questions in section B
- Show clearly all working for each question
- Mathematical tables, geometrical instruments and graph paper may be used where necessary
SECTION A (60 MARKS)
1. a) Use mathematical table, evaluate
![]()
b)Express 45.456 in form of ![]() where a and b are both integers.
where a and b are both integers.
2. a) If ![]() ,
,
evaluate ![]()
b)Solve for x the following equation 32x-3 X 8x+4 = 64 ![]() 2x
2x
c)Rationalize the denominator ![]()
3. a) Find value of P which makes the following equations perfect square
i) x2 + 8x +P=0
ii) x2 - ![]() x + P=0
x + P=0
b) Solve for x the equation ![]()
4. a)Given the universal set U={p, q, r, s, t, x, y,z} A={p, q, r, t} B={r, s, t, y }. Find i)(AUB) ii)(A’nB’)
b)In a class of 60 students, 22 students study Physics only, 25 study Biology only and 5 students study neither Physics nor Biology. Find i) Number of students study Physics and Biology. ii) Number of students that study Biology.
5. a) A, B and C are to share T.sh 120,000/= in the ratio of![]() . How much will each get?
. How much will each get?
b)A radio is sold at T. sh 40,500/= this price is 20% value added tax(V.A.T). Calculate the amount of V.A.T.
6.a) The sum of 1st n-terms of certain series is 2n-1, show that this series is Geometric Progression. Find an the nth term of this series.
b) Point P is the mid-point of a line segment AB where A(-3,8) and B(5,-2), find an equation through P which is perpendicular to AB.
7.a) Without using mathematical table, evaluate ![]()
b) A man standing on top of cliff 100m high, is in line with two buoys whose angles of depression are 170 and 210. Calculate the distance between the buoys.
8.a) The lengths of two sides of triangle are 14cm and 16cm. Find the area of the triangle if the included angle is 300.
b)The area of a regular 6-sided plot of land inscribed in a circular track of radius r is 720cm2. Find the radius of the track.
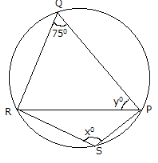
9.a) Find values of angles marked x0 and y0 in the figure below
b) Prove that exterior angle of cyclic quadrilateral is equal to interior opposite angle.
10. a)Solve for x if ![]()
b) A two-digit of positive number is such that, the product of the digits is 8. When 18 is added to the number, then the digits are reversed. Find the number.
SECTION B (40 MARKS)
11. The daily wages of one hundred men are distributed as shown below
| Wages in T.Sh. x 1,000 | 3.0-3.4 | 3.5-3.9 | 4.0-4.4 | 4.5-4.9 | 5.0-5.4 | 5.5-5.9 | 6.0-6.4 | 6.5-6.9 |
| Number of men | 4 | 6 | 10 | 14 | x | 20 | 14 | 6 |
a) Find the value of x
b) Calculate the daily mean wage of the 100 men
c) Draw histogram to represent this data and use it to estimate Mode
d) Draw cumulative frequency curve and use it to represent Median
12. Shirima makes two types of shoes A and B. He takes 3hours to make one shoe of type A and 4hours to make one shoe of type B. He works for a maximum of 120hours. It costs him sh. 400 to make a pair of type A and sh. 150 to make of type B. His total cost does not exceed sh.9000. He must make at least 8 pairs of type A and more than 12 pairs of type B.
a) Write down the inequalities that representing the given information.
b) Represent these inequalities graphically
c)Shirima makes a profit of sh. 150 on each pair of type A and sh.250 on each pair of type B. Determine the maximum possible profit he makes.
13. The following trial balance was extracted from the books of Magoma Moto at 31stt December 2015
| Name Of Account | Dr | Cr |
| Sales | 1,800,000/= | |
| Purchases | 1,155,000/= | |
| Opening Stock | 377,000/= | |
| Carriage inwards | 32,000/= | |
| Carriage outwards | 23,000/= | |
| Return Inwards | 44,000/= | |
| Return Outwards | 35,000/= | |
| Salaries and wages | 244,000/= | |
| Motor expenses | 66,000/= | |
| Rent | 45,000/= | |
| Discount allowed | 12,000/= | |
| General office expenses | 120,000/= | |
| Motor vehicles | 2,400,000/= | |
| Furniture and Fittings | 600,000/= | |
| Debtors | 457,000/= | |
| Creditors | 304,000/= | |
| Discount Received | 35,600/= | |
| Cash at bank | 387,000/= | |
| Cash in hand | 12,000/= | |
| Drawings | 205,000/= | |
| Capital | 4,005,000/= |
Stock at 31stt December 2015 was Tsh.499,000/=
a) Prepare trading, profit and loss account for the year ended 31stt December 2015
b)The balance sheet as at 31stt December 2015
14.a) In the triangle ABC below, find values of angles marked x0 and
y0 where AB=12cm, BC=7cm and AC=8cm

b) Solve the following equations given that ![]()
i) ![]()
ii) ![]()
c) Show that ![]()
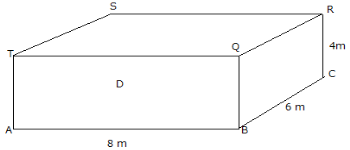
15. a) In a figure below, represents a room 8m by 6m by 4m. Calculate
i)Length of diagonal AR
ii) Angle that AR makes with the floor
iii) Angle which plane TSAD makes with plane TSBC.
b)A water pipe made of material 2cm thick has an external diameter of 16cm. Find the volume of material used in making of the pipe 200m long.
16. a) The function f is defined as follows:
F(x) = 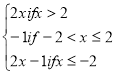
![]()
i) Sketch the graph of f(x)
ii) Determine domain and range
iii) Find i) f(1) ii) f(-4) iii) f(π)
b)For what values of x is function f(x)=![]() is undefined?
is undefined?
FORM FOUR MATHEMATICS EXAM SERIES 6
FORM FOUR MATHEMATICS EXAM SERIES 6
Hub App
 For Call,Sms&WhatsApp: 255769929722 / 255754805256
For Call,Sms&WhatsApp: 255769929722 / 255754805256
 For Call,Sms&WhatsApp: 255769929722 / 255754805256
For Call,Sms&WhatsApp: 255769929722 / 255754805256







