THE UNITED REPUBLIC OF TANZANIA NATIONAL EXAMINATIONS COUNCIL OF TANZANIA CERTIFICATE OF SECONDARY EDUCATION EXAMINATION
041 BASIC MATHEMATICS
(For Both School and Private Candidates)
Time: 3 Hours Tuesday, 06th November 2018 a.m.
Instructions
- This paper consists of sections A and B with a total of sixteen (16) questions.
- Answer all questions in section A and four (4) questions from section B. Each question in section A carries six (6) marks while each question in section B carries ten (10) marks.
- All necessary working and answers for each question done must be shown clearly.
- Mathematical tables may be used.
- Cellular phones, calculators and any unauthorized materials are not allowed in the examination room.
- Write your Examination Number on every page of your answer booklet(s).
SECTION A (60 Marks)
Answer all questions in this section.
1.If 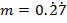 and
and 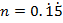 find the fraction of
find the fraction of 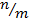 in its simplest form
in its simplest form
View Ans
(b).Find the GCF of 210, 357 and 252.
View Ans
2. (a). Evaluate log10 40,500 given that log10 2 = 0.3010 , log10 3 = 0.4771 and log10 5 = 0.6990.
View Ans
(b). Find the values of x and y if
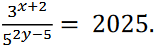
View Ans
3. (a). In a school of 60 teachers, some drink Fanta and some drink Coca-Cola. If 46 drink Fanta, 18 drink Coca-Cola and 14 drink both Coca-Cola and Fanta. How many teachers drink neither Fanta nor Coca-Cola? (Use Venn diagram)
View Ans
(b).Use the figure below to answer the following questions:
(i). Write the expression for the total area of rectangles A and B.
(ii). If the total area of rectangles A and B is 98 square centimeters, find the value of x
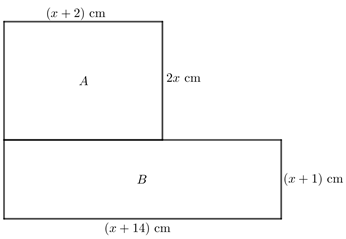
View Ans
4. (a).
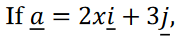
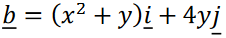
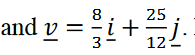
Find x and y given that
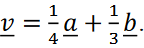
View Ans
(b). Find the point of intersection of the lines x - 2y = -5 and 2x + 7y - 34 = 0
View Ans
5. In the following figure, a regular hexagon is inscribed in a circle. If the perimeter of the hexagon is 42 cm, find:
(a) The radius of the circle.
(b) The area of the circle and the regular polygon.
(c)The area of the shaded region.
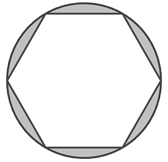
View Ans
6. (a)Mukasa received Ushs 1,000,000 from his sister in Uganda. How much did he get in Tanzanian currency (Tshs) if one Ugandan shilling was equivalent to 0.65 Tanzanian shilling?
View Ans
(b) The energy (E) stored in an elastic band varies as the square of the extension (x). When the elastic band is extended by 4 cm, the energy stored is 240 joules. What is the energy stored when the extension is 6 cm? What is the extension when the stored energy is 60 joules?
View Ans
7. (a) Three relatives shared Tshs 140,000 so that the first one got twice as much as the second, and the second got twice as much as the third. How much money did the first relative get?
View Ans
(b) Kitwana paid Tshs 900,000 for a desktop computer and sold it the following year for Tshs 720,000. Find:
- The loss made,
- The percentage loss.
View Ans
8. (a) If an arithmetic progression has A1 as the first term and d as the common difference,
- write the second, third, fourth and fifth terms.
- Establish the formula for the sum of the first five terms of the arithmetic progression by using the results in part (i).
View Ans
(b) The first and second terms of a geometric progression are 3 and 9 respectively.
- Find the third, fourth and fifth terms.
- Verify that the sum of the first 5 terms is given by
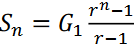
by using the results in part (i).
View Ans
9. (a) Find the distance PR in the following figure if the lines PR and RQ are perpendicular.

View Ans
(b) A flagpole is 5 meters high. Find to the nearest cm, the length of its shadow when the elevation of the sun is 600.
View Ans
10.(a) Use factorization method to solve the quadratic equation x2 ? 9x + 14 = 0.
View Ans
(b) Find the values of x that satisfies the equation
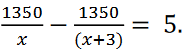
View Ans
SECTION B (40 Marks)
Answer four (4) questions from this section.
11. A farmer needs to buy up to 25 cows for a new herd. He can buy either brown cows at 50,000/= each or black cows at 80,000/= each and he can spend a total of not more than 1,580,000/=. He must have at least 9 cows of each type. On selling the cows he will make a profit of 5,000/= on each brown cow and 6,000/= on each black cow. How many of each type he should buy to maximize profit?
View Ans
12. The scores of 45 pupils in a Civics test were recorded as follows:
| 30 | 65 | 50 | 62 | 40 | 35 | 64 | 32 | 28 | 59 | 60 | 82 | 24 | 35 | 63 |
| 68 | 46 | 48 | 73 | 92 | 54 | 46 | 63 | 75 | 58 | 43 | 71 | 72 | 27 | 28 |
| 61 | 71 | 36 | 64 | 80 | 61 | 64 | 76 | 64 | 35 | 76 | 73 | 70 | 64 | 46 |
- Construct a frequency distribution table of the given data, taking equal class intervals 21 – 40, 41 – 60, …
- Calculate the mean score.
- Draw the cumulative frequency curve and use it to estimate the median.
View Ans
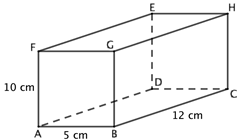
13.(a) In the following cuboid, AB = 5 cm, BC = 12 cm and BG = 10 cm. Calculate:
(i) The length of AH (give your answer correct to one decimal place).
(ii) The angle CAH.
View Ans
(b) In the following figure A, B, C and D lie on the circle; O is the centre of the circle, BD is its diameter and PAT is the tangent of the circle at A.

If angle ABD = 59 , CDB = 35, find ACD, ADB, DAT and CAO.
View Ans
14.Mwanne commenced business on 1st April, 2015 with capital in cash 200,000/=
 For Call,Sms&WhatsApp: 255769929722 / 255754805256
For Call,Sms&WhatsApp: 255769929722 / 255754805256
 For Call,Sms&WhatsApp: 255769929722 / 255754805256
For Call,Sms&WhatsApp: 255769929722 / 255754805256
 For Call,Sms&WhatsApp: 255769929722 / 255754805256
For Call,Sms&WhatsApp: 255769929722 / 255754805256